Science concepts:Vectors, vector components, defining axes (and some torque)
Mr. Miyagi had it right. You shouldn’t learn how to attack until you can guard yourself against incoming attacks. Otherwise, your fight will end up looking like one of those amateur cage fights where neither person has any regard for their own well-being and just starts swinging for the fences hoping for a lucky punch. It’s exciting to watch, but you don’t see a lot of that in the pro leagues and for good reason.
Four Levels of Defense
There are a myriad of ways to defend against attacks, but they can all be put into the following categories (in increasing order of preference):
- Blocking
- Parrying
- Evading
- Intercepting
Blocking involves taking the full force of an attack on a part of your body that can take the damage with minimal negative consequences, usually an arm, leg, or large muscle like the lats. This is the least desirable of the four, but it beats getting hit in the face.
Parrying is a more graceful version of a block. Rather than taking all of the force of the attack on your body, you redirect the attack around you. Compared to blocking, this is excellent. The damage to your body is virtually non-existent, and you may even gain an advantage through unbalancing your opponent.
Evading is Mr. Miyagi’s preferred method of defense: “no be there”. This method of defense is even better than parrying because it leaves all of your limbs available for counter-attacking.
Intercepting is Bruce Lee’s preferred method of defense, which is more along the line of “you’re not punching…I’M PUNCHING!” This involves perceiving your opponent’s intention to attack through telegraphed movements or other signs and interrupting their attack with a quicker, more efficient attack. The opponent starts first, but you finish first. This is hard to achieve, but I will address it in a later post.
The Science
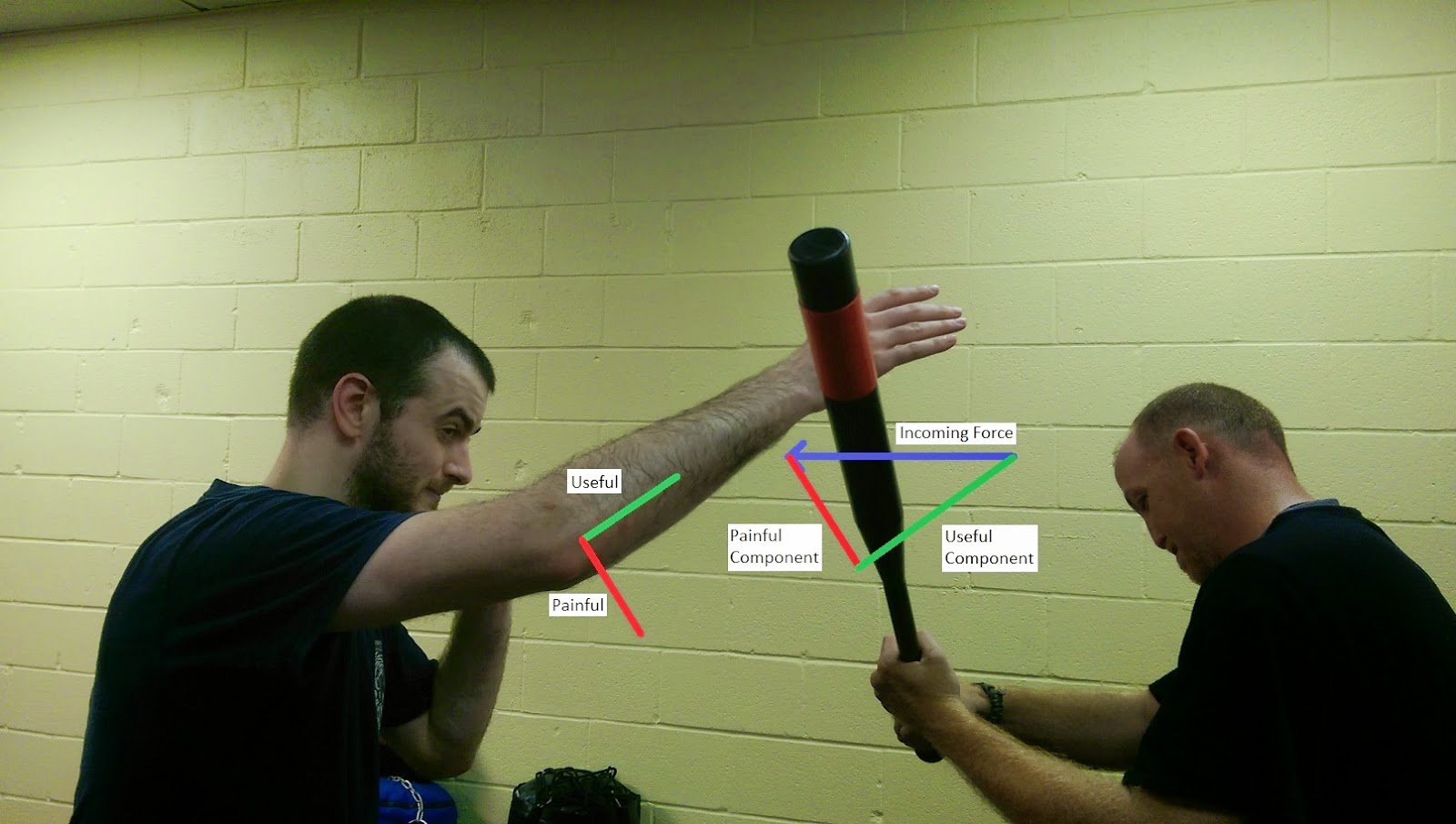
In this post, I’ll be focusing on the first two methods: blocking and parrying. Both of them involve getting your hands (or whatever) on the incoming attack before it hits something that you’d rather didn’t get hit (face, gut, groin, etc.). So, what’s the differentiator? Force vectors. The main difference between blocking and parrying has to do with the relative angle of the defensive limb’s motion to the offensive limb’s motion. To illustrate, imagine someone is swinging a baseball bat at the side of your head.
 |
| Here, Mike seems to be enjoying the prospect of smashing my face with a bat |
A block might be to put your forearm up to take the force of the attack. Sure, you can “roll with the punches”, which is a defensive tactic for reducing the maximum impulse (discussed here) of the attack, but you’re still taking a baseball bat to your forearm. If I were a betting man, I’d put my money on the bat. Now instead consider using your forearm to deflect the bat up and over your head (or down in front of your body, whichever makes more sense at the time). You didn’t stop the bat. You just prevented it from hitting your vital targets. The bat’s force redirects around you so you don’t have to absorb it.
A block meets a force head on. If the attack is coming from the left, then you push left. If it comes from the right, you push right. If it comes straight at you, you prepare to push forward into it. This is good for stopping the movement of the attack, but it also means that all of the energyis transferred into your body. There’s only so much a body can take before it breaks. Meeting hard and fast attacks head on with blocking is a good way to discover how much your body can take. I don’t recommend it if you can help it.
An ideal parry will tap an attack out of the way at a right angle (90 degrees, or an ‘L’ angle) to the attack’s motion. In the bat example, tapping the bat up would be perfect if it were swung horizontally. If instead the bat were swung straight down towards your head, then tapping it straight left or right would be ideal. So, a pure parry is 90 degrees off of a pure block. In the middle is a continuum sliding from one to the other.
Why not always use a pure and perfect parry? Let’s go back to the bat example. How confident are you that you can pop your arm straight up at just the right moment to get the bat to redirect over your head? I don’t know about you, but I’ve been practicing this stuff for years and I’m not there yet — at least not when a fast swinging bat is involved. Instead you have to choose angle of deflection as close to 90 degrees to the attack as possible while still being able to make contact with the attack, which is going to depend on a lot of things: speed, distance, timing, luck, etc.
Vectors and Axes
A key idea when operating on vectors is the idea of equivalence. Imagine simultaneously getting pushed from behind and from the left side. Which direction would you go? You’d go forward and to the right, just as if one person had pushed you from your back left side.
That’s vector equivalence. Equivalence states that multiple vectors can be added together to create a single resultant vector. This is useful for simplifying problems. Equivalence also works in reverse. If I have a single vector, which might be coming in at a funky angle that’s hard to do calculations on, then I can break it into more convenient pieces as long as those pieces add up to the original vector. We’ll do that shortly. (We’ll be doing this qualitatively so there won’t be any trigonometry involved. Sorry to disappoint you.)
I often tell the students that I tutor in physics that they need to define their axes before they solve a problem involving vectors. The same applies here. That’s one of the things I like about physics, you can choose your axes in a way to make the math easy. For the bat problem, let’s set one of the axes to be parallel to the defending arm and the other to be perpendicular to it as shown in the picture.
Labeling your axes is very important. So, let’s call the parallel axis the “useful” axis and the perpendicular axis the “painful” axis. Now, let’s take the vector of the bat’s motion and break it up into components that match up with our axes. Hopefully, the naming of the axes is a big enough hint for you. We want the angle of the block/parry to help minimize the “painful” component vector while still providing enough redirection to the bat to get it away from the head.
“Generalize and apply!”
Back at Ohio State, I took a number theory course with Professor Vitaly Bergelson. He was a great teacher that showed the class mathematical insights into things that I never would have expected to find such beautifully simple yet impactful math. He was also very brilliant and pushed the students to grow. He would write a problem on the board and have us solve it. Then he would say “generalize and apply”, expecting us to formulate a more general version of the claim and use our first proof to guide us through the more general proof. That idea of taking a concept, generalizing it, and applying the generalization in broader areas has served me well, and I advise you to do the same.
This very concept of perpendicular motion as a parry was something I realized after years of training specific defenses to specific attacks. So, I’ve done some of the generalizing for you, but that doesn’t mean that there isn’t some bigger concept that this is just a special case for.
Above I used an example of a baseball bat swinging at the side of your head. What about other swinging attacks? What about straight attacks? Take the idea and run with it. Experiment and see what you find.

I love using physics concepts in martial arts. This is definitely something I've thought about when training (though I've been out of it too long).