Science Concepts: kinetic energy, work, momentum, impulse, integrals, derivatives
“If you think Ilook bad, you should see the other guy!” This common phrase, sometimes spoken sarcastically, reflects a fundamental principle of fighting, which is the one who receives the least damage “wins”. The goals in a fight are to inflict damage and avoid taking it. There is a lot that goes into achieving those goals. What I’ll address here is the damage inflicted when a strike is landed. Sorry, no joint locks or grappling slams today. I’m going to assume, for the sake of discussion, that the attacker (whether that is you or your opponent) has landed a strike in the desired location. So, assuming that happens, if you’re dishing it out then how do you maximize the effect, and if you’re dishing it in then how do you minimize the effect? (A little forewarning: this one is going to get really nerdy.)
Harder, Better, Faster, Stronger
Daft Punk got it right. Harder, better, faster, and stronger are all unique and separate things. Speed is great, but without structure behind it, then there’s no strength in the strike (which is fine, by the way, in knife fighting). You can have structure and strength in your movement, but if you move slowly then you won’t be knocking out too many people even if you manage to hit them. A speeding feather riding a gust of wind isn’t going to hurt you and neither will a slow rolling car (assuming it doesn’t roll over you). We need both speed and strength. This is where momentum comes in…
The Science
Momentum is the product of an object’s mass and its velocity, often written p = mv. (Aside: You may be wondering why ‘p’ is used for momentum. Let’s ignore the fact that using ‘m’ would just be confusing since ‘mass’ already uses that. Newton originally used the word ‘impetus’ to describe the quality we now know as momentum. The Latin for ‘impetus’ is ‘petere’, which means “to go to; to seek.” So, ‘p’ is for ‘petere’. Etymological nerdery completed.) You might guess that I’m getting at the point that both speed and mass are important for striking. You’d be right, but that’s only a tiny part of the picture.
Momentum is necessary for striking, but consider this: simply by existing on planet Earth you have huge amounts of momentum. You have mass (some of you more than others) and are on a planet that is speeding around the sun, which is in a solar system speeding around the galaxy, which is traveling at ridiculous speeds across the universe. “Ah,” you say, “relative momentum is the key.” That’s a move in the right direction, but still not quite there. If I’m in a car that gently accelerates me up to 60 mph, then it has taken me from a relative momentum of zero (to the ground) and up to 60 mph times my mass. My momentum at the end of that is huge, but I wouldn’t say that my body was devastated from the “strike” the car gave me. Timeis important. If we change the momentum of something very quickly, then we’re talking about serious impact…or as they say in physics, “impulse.”
Impulse is the measure of the change of momentum over time. Here’s a quick derivation of the formula:
F = ma , Newton’s 2nd law of motion
F = m Dv/t , a = Dv/t by definition of acceleration (see my post on Physics)
F t = m Dv , multiplicative inverse and multiplication property of equality (ok, now I’m just being a pedantic jerk)
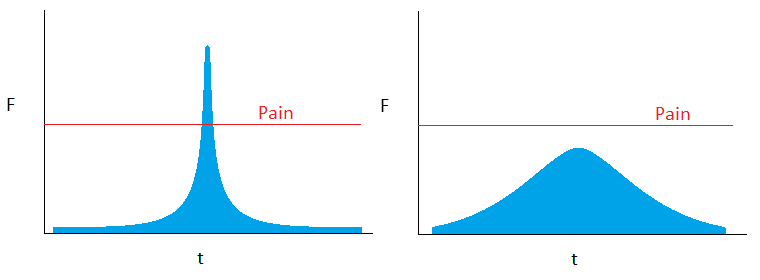
Now m Dv is the change in momentum (the Greek letter delta, ‘D’, is commonly used in math to indicate the change in a value. Think of Dv as (v2 – v1).), and that is equal to force times time. Now we are getting somewhere. If you change the momentum, say of someone’s face, by applying a large force in a little time then you can bet that it’s going to hurt. But what if the same force is applied over a larger amount of time? Depending on how much large the time interval is, it might not hurt at all. This is where the concept of “rolling with the punches” comes from. When on the receiving end of a strike, if you loosen up and move your body back with the punch so it doesn’t hit full force all at once, then the force is dissipated over a longer period of time, which reduces the maximum force you feel at any one time. Consider the following graphs.
Both graphs show force vs. time. In the graph on the left, the force is dealt in a short interval of time, spiking the graph above the pain level. Receiving this type of strike hurts. The graph on the right is an example of what happens when you roll with the punches. The blue areas of the graphs are equal (or at least they’re supposed to be…just pretend), and the area is the amount of impulse experienced. This comes from the Calculus version of the impulse equation above:
?F dt = m Dv
This almost gives us the whole picture. “There’s more?!” Yes, there’s more science, but the conclusion is worth it. Now, when someone rolls with the punch, they are applying some force to the fist. If they weren’t, then they’d just be moving backwards until the punch hit them full force. So, the force has to be dissipated a little at a time, but how should you do that exactly?
We know that the incoming strike has both mass and velocity, which means that it has kinetic energy, which is the energy an object has due to its motion. Workis when a force is applied to an object that then moves in the direction of the force. Kinetic energy and work are the final pieces of the puzzle that we need. The formula for kinetic energy is KE = ½ mv2. The formula for work is W = Fd. So, if the incoming punch has kinetic energy, then the face needs to do work to dissipate that energy. That gives us the equation W = Favgd = ½ mv2. To stopthe incoming strike, the guy rolling with the punch will need to provide an average force over a distance d. You could also think of the average force as the impact force felt by the face (remember that every action has an equal but opposite reaction). The greater the distance is the smaller that average force can be. So, impulse told us that we need to increase the impact time and the energy equations told us that we need to increase the impact distance (to avoid taking damage, that is). Those two conclusions both being true isn’t all that surprising. What is interesting about the energy equation is this: when striking, velocity is a much bigger factor than mass. I can say this because of the squared velocity. Let’s consider a qualitative example.
Suppose puncher A has a mass m and puncher B has a mass m/2. Also suppose that puncher A punches at v m/s and that puncher B punches at 2v m/s. So, puncher B has half the mass, but twice the velocity. The momentum equation will tell us that both punchers have the same momentum on their punches. However, the energy equations tell a more complete story. The punch from A has a kinetic energy of KEA = ½ mv2 . The punch from B has a kinetic energy of is
KEB = ½ (m/2)(2v)2 = (m/4)(4v2) = mv2 = 2KEA. So, B’s punch has twice the kinetic energy of A’s punch, which means that someone will need twice the distance to “roll with the punch” or they’ll just have to feel twice the average impact force. This explains why Bruce Lee had such devastating strikes despite his small size.
Conclusion
There was a lot of math and physics getting here, but the results were quite useful. First, we noted that both mass and velocity matter when striking. In particular, we want to change the momentum of our target in as little time as possible. If we’re getting hit, then we want the impact to last as long as possible. Finally, based on the energy equations, we saw that even though mass and velocity matter in striking, velocity matters more. So, when you’re practicing, learn good form to have structure that will allow you to use the mass of your body in your strikes and from there … get faster!